The mathematics of patterns
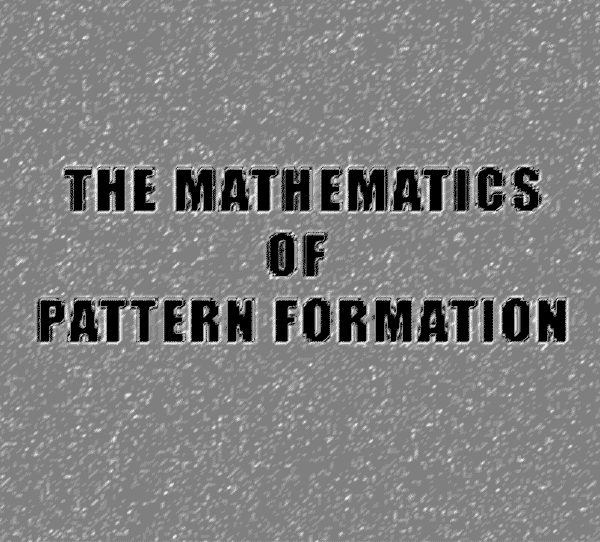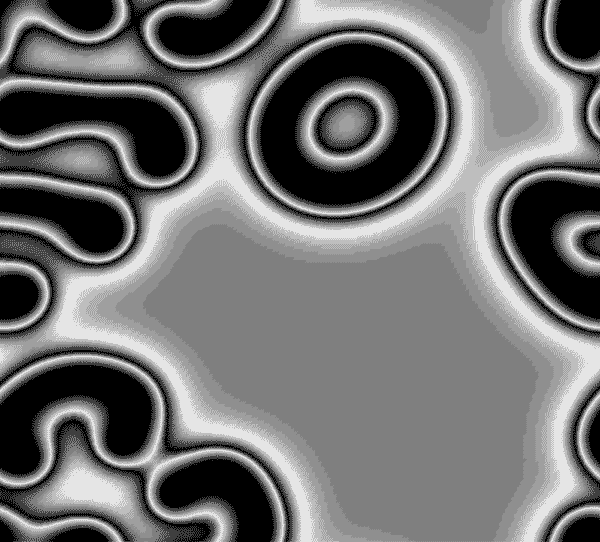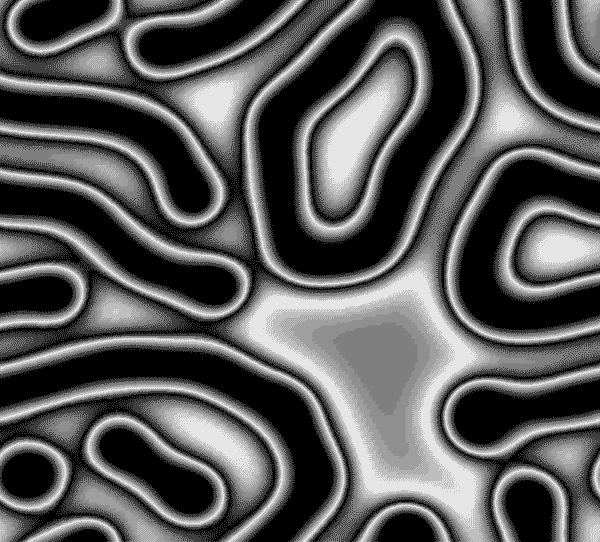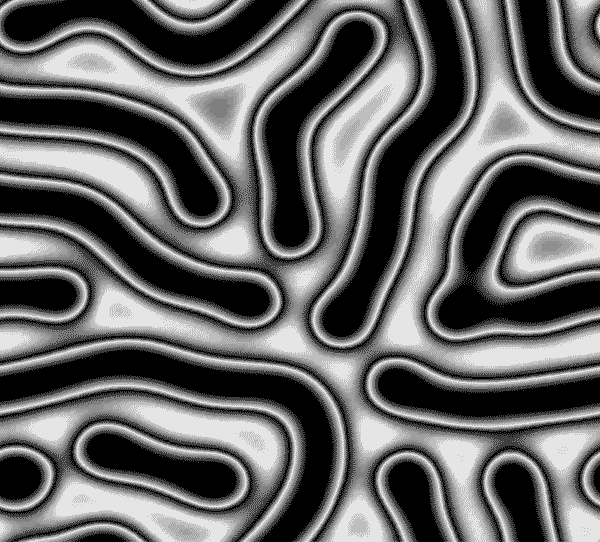
This image is produced by modeling the reaction and diffusion of two chemical concentrations, for which one is originally set to be highly concentrated in certain regions to produce the lettering shown. Dark areas correspond to high concentration of one of the species. Eventually, the dynamics of the two species are such that stripped patterns are seen.
If you look around to your surroundings, you will see patterns every which way. Maybe you will see a patterns in the alternating dark and light grain of your wooden desk or chair. Or if you are reading this while sitting outside, you might see pattern in the way tree branches, on the organization of the leaves, or on the design of a snail's shell. Beautiful and intricate patterns arise not only in nature, but also in the geometric patterns of man-made art, such as in a woven rug or a tiled mosaic.

These notes are intended to introduce you to the mathematics behind pattern formation. The first section is just a brief discussion of why we might be interested in understanding patterns in biological systems (e.g. developmental biology). The second section talks about the basic partial differential equations from which we can build many of the patterns we encounter in nature. The next sections will talk about pattern formation itself, and we will work through some interesting examples that can be applied to animal coat patterns.
Previous exposure to differential equations will make the material much easier to follow, but even this is not necessary, as all the concepts are supplemented with illustrations and animations in order to give you the big picture.
This project is an undergraduate entry for the DSWeb2013 Contest for Teaching Dynamical Systems, and is also a portion of the author's independent work for the Certificate program of the Program in Applied and Computational Mathematics at Princeton.
Princeton University